One of the most important concepts of Structures of Equality (SoE), and what sets it apart from other word problem strategies, is the math main idea. 

Whether you’re a teacher, a parent, or a student, you’ve likely experienced the same struggle when it comes to word problems: comprehension. For decades, this has been an issue. Students don’t understand what the problem is asking and they resort to tips and tricks instead of meaningful strategies.

This makes it hard to tease out where misconceptions occur. Is it the understanding of the number story itself or is it the arithmetic? Without the use of visual models where students show their thinking, it’s even harder to pinpoint.

This blog explores how to use:

• the math main idea
• the Repeated Equal Groups (REG) structure
• Math Practice Standard 2: Reason Abstractly and Quantitatively

to help students transition between abstract reasoning and real-world problem-solving. I’ll also share how creating number stories deepens their understanding.

Understanding problems that lend themselves to the REG model are crucial as the mathematics behind them is foundational for understanding multiplication, division, and proportional reasoning.

The Math Main Idea: Repeated Equal Groups

The REG structure is used when the math main idea of a number story is composing equal groups or parts to form a total or decomposing a total into equal groups or parts. This idea is essential for understanding concepts like:

• Multiplication: How many groups of a certain size make up the total?
• Division: How many items are in each group, or how many groups are needed?

By identifying this math main idea, students learn to recognize patterns and apply their understanding to various contexts.

Example: Repeated Equal Groups in Word Problems

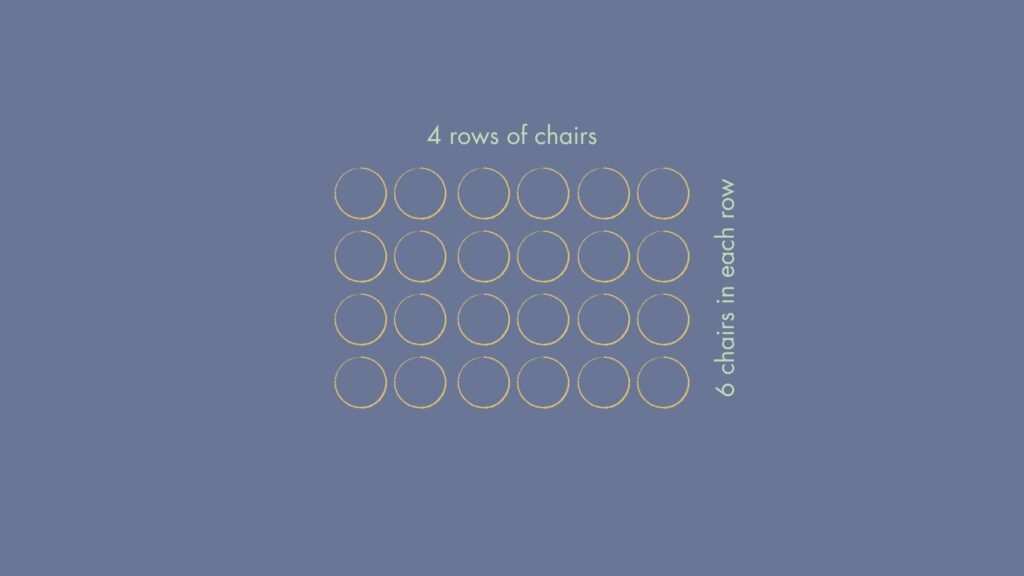
• Number Story: A teacher is setting up chairs for 4 rows. Each row has 6 chairs. How many chairs are there?
• Math Main Idea: composing equal groups of chairs to find the total number of chairs (4 groups or rows of chairs with 6 chairs in each row)
• REG Structure:

Extending Understanding: Create Your Own Number Stories

Once students are comfortable solving problems using the Repeated Equal Groups structure, another way to connect literacy and mathematics is to challenge them to create their own number stories. This encourages them to reason both abstractly and quantitatively, aligning with Math Practice Standard 2.

Example Activity

1. Use a structure students have already drawn and ask them to come up with other stories that could represent the same model. I like to incorporate partner talk here and prompt students with: Tell your partner another story that matches this structure.

For example, if you were to continue with the structure modeled above, here are alternative number stories students might create:

• A farmer has 4 hens. Each hen lays 6 eggs a day. How many eggs will the farmer collect each day?
• Nadia is inviting 6 friends to her birthday party. If she wants to give each friend 4 items in their goody bags, how many items will she need to buy?

Although SoE is focused around comprehension and not solving, you could extend the activity to include arithmetic and ask the students to write expressions or equations to represent their number stories.

2. If students already came up with a solution and know the total is 24, you can include all the values in the REG model and encourage them to come up with number stories that represent the total being decomposed into equal groups or parts. Here are some examples:

• Mr. Smith bought 24 new books for the classroom library. If he has 6 baskets and splits them equally, how many books will go in each basket?
• Jaden and his mom baked a batch of 24 cookies. If there are 4 people in their family and they share the cookies equally, how many cookies will each person get?

For each number story, ask students to show where their values are represented 

in the structure and how they would label them. 

3. Discuss and Reflect 

• Compare the students’ stories.
• Discuss how the structure itself remains consistent across different contexts because, in all contexts, the math main idea describes composing equal parts to form a total or decomposing a total into equal parts.
• Note how the values remained the same but the labels changed depending on the context. Pay particular attention to which numbers represent the groups and which represent how many in each group.

Connecting to Math Practice Standard 2

This activity reinforces the two key components of Math Practice Standard 2:

1. Decontextualization:
   • Students abstract the story into a mathematical model or equation, such as 4 x 6 or 24 ÷ 4.
2. Contextualization:
   • They interpret the abstract model back into a meaningful real-world scenario, ensuring the math main idea aligns.

This back-and-forth process strengthens their ability to think flexibly and connect abstract math to practical applications.

Why Focus on the Math Main Idea?

Highlighting the math main idea supports students in:

• Recognizing patterns and structures: They see that many problems share the same underlying relationships.
• Reasoning deeply: By focusing on the structure, students go beyond rote memorization and engage with the meaning of operations.
• Building real-world connections: They understand how math describes situations they encounter in daily life.

Bringing It All Together

Here’s how you can integrate the REG structure, the math main idea, and Math Practice Standard 2 into your classroom:

1. Introduce the Math Main Idea
   • Start each problem by explicitly identifying the math main idea.
2. Model with Visuals
   • Have students represent the math main idea with its corresponding structure. Remember, some students may choose to represent REG problems with the Parts Equal Total (PET) structure. Read about why that’s okay.
3. Encourage Story Creation
   • Provide mathematical representations and challenge students to write their own number stories that match the structure.
   • If students do this for other structures as well, you could collect the stories and plug them into the fillable math main idea sorting mat for a future activity.
4. Reflect on Connections
   • Discuss how their stories illustrate the math main idea and how they transitioned between abstract and contextual thinking.

Conclusion

The Repeated Equal Groups structure is used to represent an important math main idea that builds foundational skills in multiplication and division. By connecting this structure to Math Practice Standard 2, we equip students to think abstractly, reason quantitatively, and see math as a tool for solving real-world problems.

Encourage your students to create their own number stories and watch their comprehension of number stories and the underlying mathematical concepts deepen.