October 25, 2023
Updated 8.13.2025
We recently looked at how keywords can cause confusion when students are given a problem with a comparison situation. Today, you’ll see two word problem strategies side by side: CUBES, a procedural method, and Structures of Equality (SoE), a framework for comprehension.
Here are 3 ideas to consider while you look at the examples:
- Do students have to use critical thinking to engage in the process?
- Is it possible to solve the problem without understanding what it’s asking?
- Will this strategy work every time?
CUBES and SoE: an overview
CUBES
This is a procedural method that relies on completing a series of steps to arrive at a solution.
The steps:
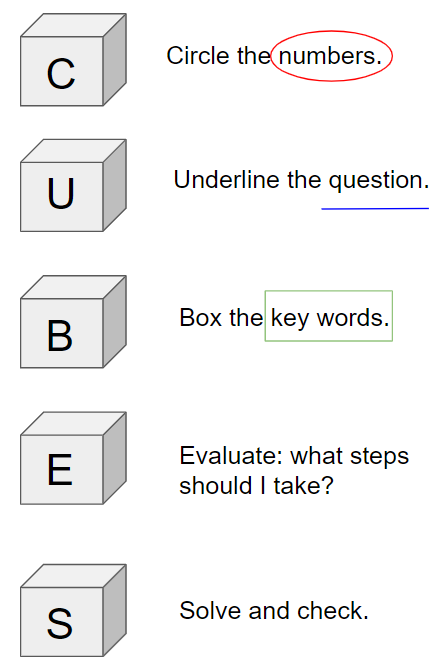
To find key words, there’s often a chart to reference:

SoE
The structures are visual representations of the math main idea of a story. It’s a framework that focuses on students understanding what’s happening in a word problem.
Let’s look at one word problem and show how students would use each approach to model it.
Felix had 6 chocolates. His mom gave him 3 more. How many chocolates does Felix have now?
Cube

Parts Equal Total SoE
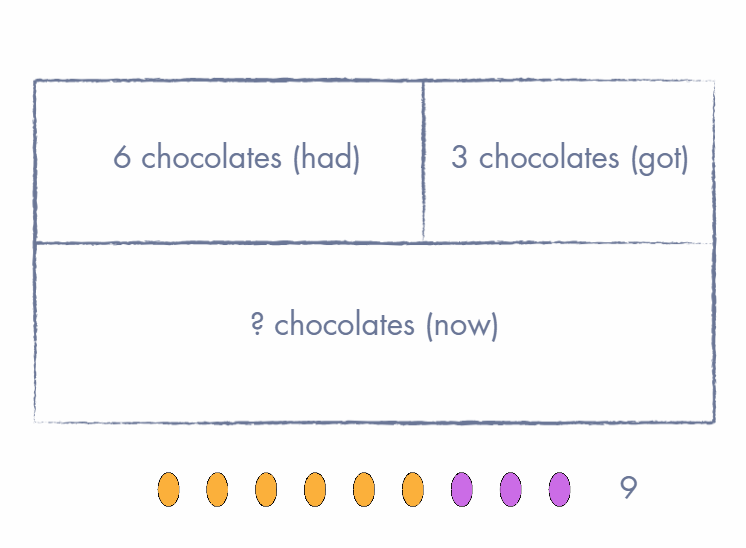
We arrive at the same solution with both approaches. However, using CUBES was a rote process. We didn’t have to engage in any thinking. We just had to follow a series of steps to arrive at an expression we could use to solve. This is problematic because it’s not a series of steps that will work every time.
With SoE, students have to comprehend that:
- We have two parts, 6 and 3.
- Each number represented a quantity with a label.
- The total value would be equal to the parts.
- The math main idea of this story describes parts being composed to form a total or a total being decomposed into parts.
- We have two parts, 6 and 3.
- Each number represented a quantity with a label.
- The total value would be equal to the parts.
This focus on the situation as it’s occurring in the story and understanding the relationship on the values leads to comprehension every time.
Now, let’s try a different type of problem, one in which we’re looking for the difference.
Kianna had 6 chocolates. Mia had 3 chocolates. How much more chocolate did Kianna have than Mia?
Cubes:

Compare SoE

In this case, the student who used CUBES followed the steps, but the steps didn’t lead to an expression that matches the situation in the story problem. They are supposed to evaluate, then solve and check. But with this method, there’s no way to tell if the solution makes sense or not.
With the Compare SoE, the student had to comprehend the problem in order to visually represent it correctly. They understood:
- The math main idea of this story describes comparing two distinct sets or quantities.
- We had a larger value (Kianna’s chocolate) and a smaller value (Mia’s chocolate).
- Mia’s chocolate was equivalent to part of Kianna’s chocolate, which they represented with a line of equality.
- In order to figure out the difference, or the “more”, they need to see that Kianna’s 6 chocolates can be decomposed into the part that is equal to the 3 chocolates Mia has and the more.
To solve, the student who used a SoE could use the equation 3 + ___ = 6, or the expression 6 – 3.
A comparsion of methods
CUBES is a step-by-step method. It works sometimes. When students attempt to solve word problems as a series of steps to complete, they focus on procedures rather than conceptual understanding. This is why so many teachers get frustrated when teaching word problems. And why so many students don’t understand what the problem is asking them to do.
SoE are not a series of rote steps or procedures. The structures use a systematic approach that helps students comprehend word problems so they can successfully choose strategies and operations to help them solve problems accurately.
- They have to use critical thinking to engage in the process.
- They must show understanding to complete the visual representation.
- SoE work every time. All of the problem types in the K-5 Common Core standards can be represented with one of the three structures: Parts Equal Total, Repeated Equal Groups, or Compare.
Conclusion
SoE provide a systematic framework that encourages critical thinking which benefits both students and teachers. By grasping the understanding of how to translate story problems into visual representations, problem-solving becomes more accessible. Students comprehend what is happening within the problems and engage the critical thinking skills necessary for continued success with mathematics.