If you ask a first-grade teacher what they wish kids already knew in math, “math facts to ten” is probably high on the list. And if you ask middle school teachers? A lot of them would say the same. That’s because being able to easily compose and decompose numbers to make ten is critical for working within our base-ten system, which relies heavily on place value.

Memorizing facts to ten is helpful, but understanding them and building fluency is even more important. When students learn these facts in a flexible way, they build a strong foundation that makes it easier to tackle more advanced math concepts later on. 

The Erikson Institute’s Early Math Collaborative puts it this way: “An understanding of the parts-whole relationship within a set is a necessary foundation for operating on and with numbers.”

One way to develop this understanding is by using the Parts Equal Total (PET) structure. PET is a great tool for fluency because it highlights the relationship between numbers rather than treating facts as isolated pieces of information. Students can see patterns in how numbers combine and break apart, helping them move beyond memorization to real number sense.

Here’s how PET can help students really get their facts to ten. (If you’re not familiar with PET, you can learn more about it here.)

Using PET in context

First, let’s look at how this could work in a story problem before transitioning to a quick daily warm-up activity.

Example Problem:
There were 6 book baskets in the reading corner. The teacher added a few more. Now there are 10 book baskets in the reading corner. How many baskets did the teacher add?

The math main idea in this problem is two parts being composed to form a total, so this would be best represented with a PET structure.

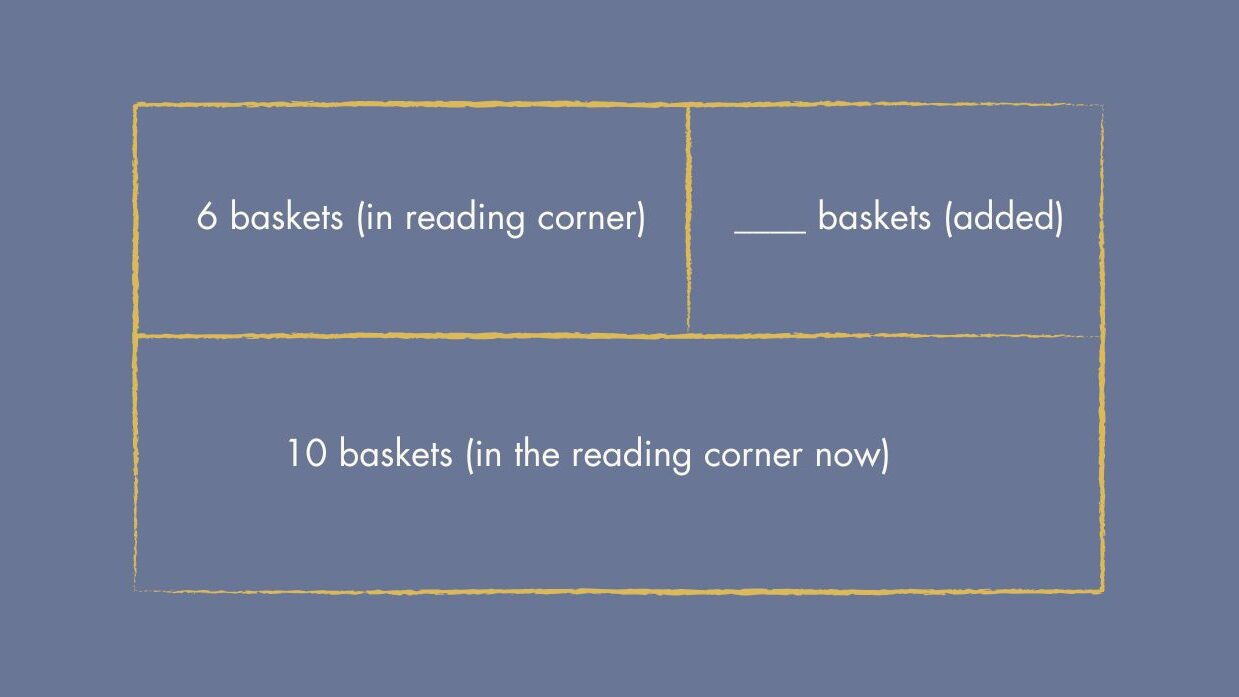
Once students have the visual model drawn, they can solve for the unknown value using any strategy they’ve learned. They would find that 4 baskets were added to the reading corner.

Building fluency with a PET warm-up

Once kids have worked with PET in a real-world problem, you can start using it as a quick daily warm-up to reinforce their number sense. This helps them see number facts as relationships rather than memorization drills.

Example:

• Show a PET structure with labels and values, but remove the number story.
• Say: “Yesterday, we figured out that when we had a total of 10 books and one part was 6 books, the other part was 4 books. What if instead of 6 books, this part was 7 books?”

Because students already worked with the model in context, they can now focus on number relationships. You can continue this process by changing which part of the PET model is unknown each day. For example:

• If this part is 2, what does the other part represent?
• If this part is 9 and the other part is 1, what is the total?

Over time, students internalize the flexibility of numbers within a PET model. This allows them to see number facts as interchangeable rather than fixed answers for procedural recall.

Ideas to make it interactive

• Have sentence strips pre-cut for students to place under the total bar of 10 for students to match, like a puzzle. For example, if the value is one part was 6, they could find the pre-cut strip that would represent 4.
• Encourage students to come up with their own number stories based on the values and labels in the PET model.
• Use whiteboards so students can quickly adjust their answers.
• Pair students up and have them quiz each other by covering one part of the PET model and asking their partner to solve for the unknown value.

Why this works

When kids use PET in both word problems and quick exercises, they start seeing patterns in how numbers can be composed and decomposed. Instead of viewing facts to ten as random numbers to memorize, they recognize how numbers work together.

That makes it way easier to build on more advanced strategies, like:

• Break apart to make ten (example: solving 8 + 6 by breaking apart 6 into 2 and 4 to make 10 + 4).
• Regrouping in addition and subtraction
• Making connections to subtraction (If 6 + 4 = 10, then 10 – 6 = 4)

Instead of treating math facts like something to drill, PET ties them into real problem-solving—so kids actually understand numbers, not just memorize them.